|
The two scales on the back of the slide marked S and T are the
sine and tangent scales respectively.
|
|
|
|
Sines
|
|
|
 The S
scale is divided to read in degrees and minutes from about
0°34′ to 90°. It is designed to be used with the
A and B scales. The S
scale is divided to read in degrees and minutes from about
0°34′ to 90°. It is designed to be used with the
A and B scales.
|
|
|
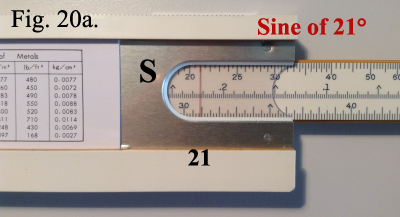
 Example:
Find the sine of 21°. Example:
Find the sine of 21°.
|
|
|
 Set the
slide to bring 21 on the S scale under the right-hand fixed
hairline on the back of the rule. Example shown in Figure 20a.
Turn the rule over, and read
358 on the B scale under the right index of the A scale.
Example shown in Figure 20b.Note that the values of sines found
on the right-hand half of the A and B scales range from 0.1 to
1.0 and those on the left-hand half range from 0.01 to 0.1.
Therefore, our answer in this example is 0.358. Set the
slide to bring 21 on the S scale under the right-hand fixed
hairline on the back of the rule. Example shown in Figure 20a.
Turn the rule over, and read
358 on the B scale under the right index of the A scale.
Example shown in Figure 20b.Note that the values of sines found
on the right-hand half of the A and B scales range from 0.1 to
1.0 and those on the left-hand half range from 0.01 to 0.1.
Therefore, our answer in this example is 0.358.
|
|
|
 When
given the sine, the procedure is reversed to find the angle. When
given the sine, the procedure is reversed to find the angle.
|
|
|
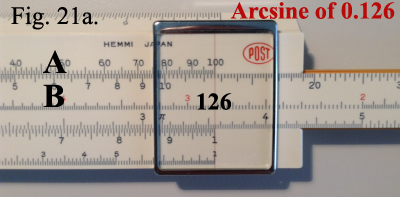
 Example:
Find the angle whose sine is 0.126. Example:
Find the angle whose sine is 0.126.
|
|
|
 Since the
sine is greater than 0.1, 0.126 is located on the right-hand
segment of the B scale. Draw the slide to bring 126 on the
right half of the A scale. Example shown in Figure 21a.
Turn the rule over, and read the angle
7°15′ on the S scale under the fixed hairline.
Example shown in Figure 21b. Since the
sine is greater than 0.1, 0.126 is located on the right-hand
segment of the B scale. Draw the slide to bring 126 on the
right half of the A scale. Example shown in Figure 21a.
Turn the rule over, and read the angle
7°15′ on the S scale under the fixed hairline.
Example shown in Figure 21b.
|
|
|
|
Alternate Method (Sines)
|
|
|
 Remove the
slide from the rule and re-insert so that the S, L en T scales
are face up. With the slide centered so that the indices of the
A scale line up with the ends of the S scale, sines can be read
on the A scale directly opposite any angle on the S scale. Thus,
to find the value of sin 7°, set the cursor hairline over 7 on
the S scale and read the answer 0.122 under the hairline on the
A scale. Example shown in Figure 22. Remove the
slide from the rule and re-insert so that the S, L en T scales
are face up. With the slide centered so that the indices of the
A scale line up with the ends of the S scale, sines can be read
on the A scale directly opposite any angle on the S scale. Thus,
to find the value of sin 7°, set the cursor hairline over 7 on
the S scale and read the answer 0.122 under the hairline on the
A scale. Example shown in Figure 22.
|
|
|
|
Tangents
|
|
|
 The T scale is divided in degrees
and minutes from about 5°45′ to 45°. It is designed to be used with the
C and D scales. The T scale is divided in degrees
and minutes from about 5°45′ to 45°. It is designed to be used with the
C and D scales.
|
|
|
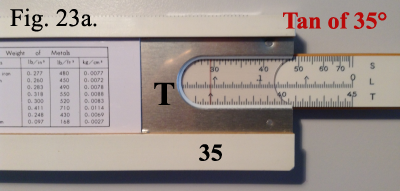
 Example: Find the value of tan 35°. Example: Find the value of tan 35°.
|
|
|
 Set 35 on the T scale under the
right-hand fixed hairline on the back of the rule. Example shown in Figure 23a.
Turn the rule over and read the answer 0.7000 on the C scale over the right index of the D scale.
Example shown in Figure 23b.
Note that the values of tangents
of angles from 5°45′ to 45° range from 0.1 to 1.0. Set 35 on the T scale under the
right-hand fixed hairline on the back of the rule. Example shown in Figure 23a.
Turn the rule over and read the answer 0.7000 on the C scale over the right index of the D scale.
Example shown in Figure 23b.
Note that the values of tangents
of angles from 5°45′ to 45° range from 0.1 to 1.0.
|
|
|
|
Alternate Method (Tangents)
|
|
|
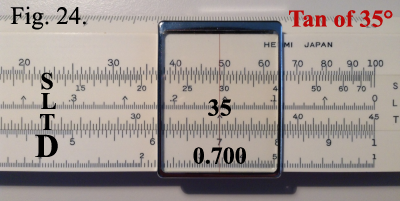
 Turn the slide rule over as
described in the alternate method for using the S scale and read directly from the
T scale to the D scale or vice versa.
Thus, to find the value of tan 35°, set the cursor hairline over 35 on the T scale
and read the answer 0.007 under the hairline on the D scale. Example shown in Figure 24. Turn the slide rule over as
described in the alternate method for using the S scale and read directly from the
T scale to the D scale or vice versa.
Thus, to find the value of tan 35°, set the cursor hairline over 35 on the T scale
and read the answer 0.007 under the hairline on the D scale. Example shown in Figure 24.
|
|
|
|
Tangents of Angles from 0°34′ to 5°45′
|
|
|
 The values of tangents and sines of
angels smaller than 5°45′ are so nearly alike that they may be considered identical
for slide rule computations. Consequently, for angles from 0°34′ to 5°45′,
tangents can be read directly using the S scale and the left half of the A or B scales,
as described in the section of sines. The values of tangents and sines of
angels smaller than 5°45′ are so nearly alike that they may be considered identical
for slide rule computations. Consequently, for angles from 0°34′ to 5°45′,
tangents can be read directly using the S scale and the left half of the A or B scales,
as described in the section of sines.
|
|
|
|
Tangents of Angles greater than 45°
|
|
|
 Using the relationship Using the relationship
|
|
|
|
|
|
|
|
tangents for angles greater than 45° can be directly computed on the slide rule.
|
|
|
 Example: Find tan 69°. Example: Find tan 69°.
|
|
|
 Solution: 90 – 69 = 21.
Set 21 on the T scale under the right-hand fixed hairline. Example shown in Figure 25a.
The answer, which is the reciprocal of tan 21, is read directly on the D scale under the
left index of the C scale as 2.605. Example shown in Figure 25b.
Note that for angles between 45° and approximately 84° the value of the tangent
ranges from 1 to 10. Solution: 90 – 69 = 21.
Set 21 on the T scale under the right-hand fixed hairline. Example shown in Figure 25a.
The answer, which is the reciprocal of tan 21, is read directly on the D scale under the
left index of the C scale as 2.605. Example shown in Figure 25b.
Note that for angles between 45° and approximately 84° the value of the tangent
ranges from 1 to 10.
|
|
|
 When in the above relationship 90 – x
is less than 5°45′, the left half of the S scale is used in making the computation.
As pointed out above, the sines and tangents of small angles may be considered equal for slide
rule purposes. When in the above relationship 90 – x
is less than 5°45′, the left half of the S scale is used in making the computation.
As pointed out above, the sines and tangents of small angles may be considered equal for slide
rule purposes.
|
|
|
 Example: Find tan 88°. Example: Find tan 88°.
|
|
|
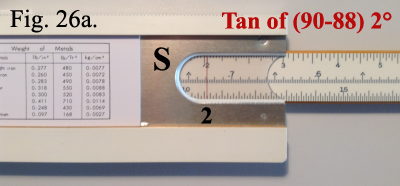
 Set 2 on the S scale (90 – 88) under
the right-hand fixed hairline. Example shown in Figure 26a.
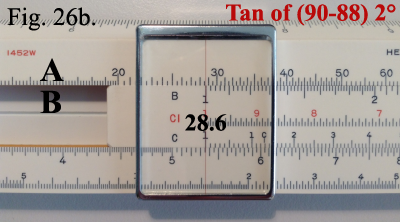
Turn the slide rule over and read the answer 28.6 on the A scale over the left index of the B
scale. Example shown in Figure 26b.
Using this method, tangents of angles up to 89°25′ can be read directly. Set 2 on the S scale (90 – 88) under
the right-hand fixed hairline. Example shown in Figure 26a.
Turn the slide rule over and read the answer 28.6 on the A scale over the left index of the B
scale. Example shown in Figure 26b.
Using this method, tangents of angles up to 89°25′ can be read directly.
|
|
|
|
Sines and Tangents of Very Small Angles
|
|
|
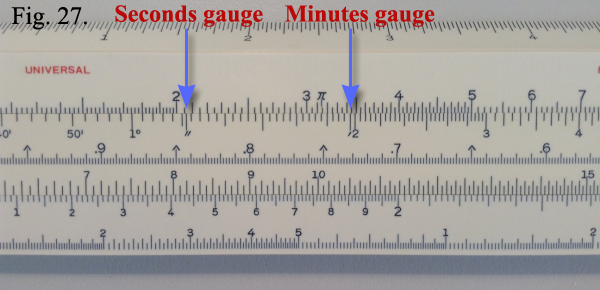
 For determining the sine or tangent
of a very small angle, two gauge points are provided on the S scale. The one identified by
the symbol (′) is called the minutes gauge point and is fount next to the 2° mark
on the S scale. The second gauge point marked (″) is found near the 1°10′
mark on the S scale. Example shown in Figure 27. They represent the value of the angle in
radians. Their use is based on the fact that for very small angles, sin x = tan x = x
(in radians), approximately. For determining the sine or tangent
of a very small angle, two gauge points are provided on the S scale. The one identified by
the symbol (′) is called the minutes gauge point and is fount next to the 2° mark
on the S scale. The second gauge point marked (″) is found near the 1°10′
mark on the S scale. Example shown in Figure 27. They represent the value of the angle in
radians. Their use is based on the fact that for very small angles, sin x = tan x = x
(in radians), approximately.
|
|
|
 Example: Find sin 3′. Example: Find sin 3′.
|
|
|
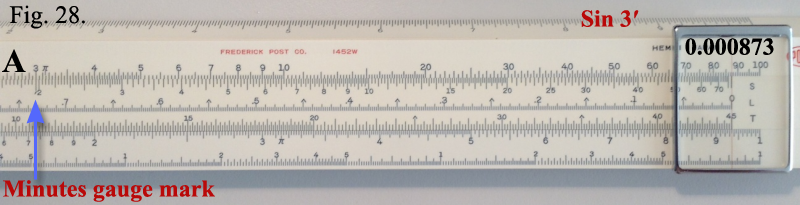
 Since sin 3′ = 3′ (in radians),
solve 3 × 1′ (in radians). The procedure when using these gauge points is the same
as the use of the CI and D scale combination for multiplication. With the slide rule turned
so that the trig scales are face up, set the minutes gauge mark under the 3 on the A scale.
Over the right index on the S scale, read 0.000873 on the A scale. Example shown in Figure 28. Since sin 3′ = 3′ (in radians),
solve 3 × 1′ (in radians). The procedure when using these gauge points is the same
as the use of the CI and D scale combination for multiplication. With the slide rule turned
so that the trig scales are face up, set the minutes gauge mark under the 3 on the A scale.
Over the right index on the S scale, read 0.000873 on the A scale. Example shown in Figure 28.
|
|
|
 Example: Find sin 3″. Example: Find sin 3″.
|
|
|
 Set the second gauge mark under 3
on the A scale. Over the left index on the S scale, read 0.0000145 on the A scale.
Example shown in Figure 29. Set the second gauge mark under 3
on the A scale. Over the left index on the S scale, read 0.0000145 on the A scale.
Example shown in Figure 29.
|
|
|
 The decimal point in the above
examples is located by noting that: The decimal point in the above
examples is located by noting that:
|
|
|
 1′ – 0.0003 radians (approximately) 1′ – 0.0003 radians (approximately)
|
 1″ – 0.000005 radians (approximately) 1″ – 0.000005 radians (approximately)
|
|