|
Reading the Scales
|
|
|
 The C and D scales are most
commonly used for multiplication and division. These scales have identical
graduations and appear on all slide rules. The C and D scales are most
commonly used for multiplication and division. These scales have identical
graduations and appear on all slide rules.
|
|
|
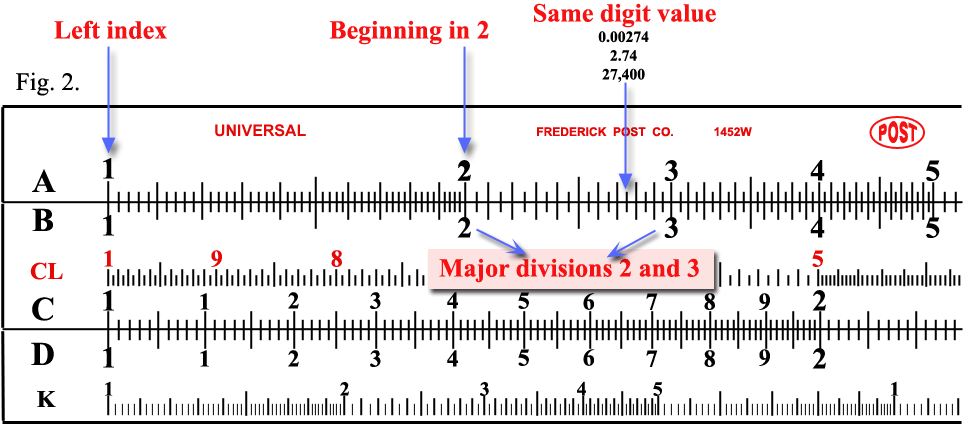
 Their left and right-hand ends
marked with the numeral ‘‘1’’, and are called left index
and right index, respectively. The major divisions are marked from 2 through 9.
Each major division is further divided into tenths. More complete divisions are given
for values between 1 and 2, covering the left-hand end of the scale. This section is
further sub-divided into ten major sub-divisions from 1 to 9, representing the
values 11 to 19 (on the POST four inch rule, only 15 mark is numbered, due to lack
of space). The tenths are still further sub-divided into tenths, fifths or halves,
as space permits. Their left and right-hand ends
marked with the numeral ‘‘1’’, and are called left index
and right index, respectively. The major divisions are marked from 2 through 9.
Each major division is further divided into tenths. More complete divisions are given
for values between 1 and 2, covering the left-hand end of the scale. This section is
further sub-divided into ten major sub-divisions from 1 to 9, representing the
values 11 to 19 (on the POST four inch rule, only 15 mark is numbered, due to lack
of space). The tenths are still further sub-divided into tenths, fifths or halves,
as space permits.
|
|
|
 On the previous page, you will
find that Figure 1 (10 inch rule) illustrate how to read various values on a typical
slide rule scale. Familiarize yourself with the scale so you will know how to find
these examples. On the previous page, you will
find that Figure 1 (10 inch rule) illustrate how to read various values on a typical
slide rule scale. Familiarize yourself with the scale so you will know how to find
these examples.
|
|
|
|
First Digit is Guide
|
|
|
 Note that all numbers that begin
with the numeral 1, regardless of the position of the decimal point, are found
somewhere between the left index and the major division 2. This space covers approximately
the left-hand third of the entire scale. All numbers beginning in 2 are found between
the major divisions 2 and 3, numbers beginning in 3 between major divisions 3 and 4,
and so on. Note that all numbers that begin
with the numeral 1, regardless of the position of the decimal point, are found
somewhere between the left index and the major division 2. This space covers approximately
the left-hand third of the entire scale. All numbers beginning in 2 are found between
the major divisions 2 and 3, numbers beginning in 3 between major divisions 3 and 4,
and so on.
|
|
|
 When it is necessary to read a
scale at a point between two graduations, the setting must be estimated to the nearest
fraction of a division. When it is necessary to read a
scale at a point between two graduations, the setting must be estimated to the nearest
fraction of a division.
|
|
|
 All numbers that have the same
digit value (e.g. 0.00274, 2.74, or 27,400) are found at the same point on the slide
rule scale, regardless of the position of the decimal point. Example shown in Figure 2. All numbers that have the same
digit value (e.g. 0.00274, 2.74, or 27,400) are found at the same point on the slide
rule scale, regardless of the position of the decimal point. Example shown in Figure 2.
|
|